April 13, 2024

[PDF] Integration Formulas for Class 12

by Bina singhDecember 7, 2022January 14, 2023

Integration is the inverse process of differentiation. In differentiation, we are given a function and asked to determine its differential coefficient or derivative. In integration, we are given the derivative of a function and asked to find that function.

Here, fundamental integration formulas for various functions are mentioned. In addition to the fundamental integration formulas, this article also provides a classification of integral formulas.

Integration formulas for class 12

Contents

Some Standard Elementary Integrals

Integration Formulas

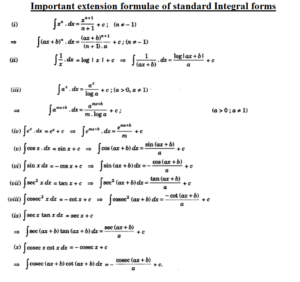
Important extension formulae of standard Integral forms

Integrals of some special functions

(i) $\large \int \frac{dx}{x^{2}-a^{2}}=\frac{1}{2a}log\left | \frac{x-a}{x+a} \right |+c$

(ii) $\large \int \frac{dx}{a^{2}-x^{2}}=\frac{1}{2a}log\left | \frac{a+x}{a-x} \right |+c$

(iii) $\large \int \frac{dx}{x^{2}+a^{2}}=\frac{1}{a} tan^{-1}\frac{x}{a}+c$

(iv) $\large \int \frac{dx}{\sqrt{x^{2}-a^{2}}} =log\left | x+ \sqrt{x^{2}-a^{2}} \right |+c$

(v) $\large \int \frac{dx}{\sqrt{a^{2}-x^{2}}} = sin^{-1}\frac{x}{a}+c$

(vi) $\large \int \frac{dx}{\sqrt{x^{2}+a^{2}}}=log\left | x+ \sqrt{x^{2}+a^{2}}\right |+c$

(vii) $\large \int \frac{dx}{x\sqrt{x^{2}-a^{2}}} =\frac{1}{a}sec^{-1}\frac{x}{a}+c$

(viii) $\large \int tanx \, dx = log \left | secx \right | +c$

(ix) $\large \int cot x \, dx = log \left | sinx \right | +c$

(x) $\large \int secx \, dx = log \left | (secx +tanx)\right | +c =log \left | tan(\frac{\Pi }{4}+\frac{x}{2}) \right |+c$

(xi) $\large \int cosecx \, dx = log \left | (cosecx - cotx)\right | +c =log \left | tan\frac{x}{2} \right |+c$

(xii) $\large \int e^{ax}sinbxdx=\frac{e^{ax}}{a^{2}+b^{2}} \left ( asinbx-bcosbx \right )+c$

(xiii) $\large \int e^{ax}cosbxdx=\frac{e^{ax}}{a^{2}+b^{2}} \left ( acosbx-bsinbx \right )+c$

(xiv) $\large \int \sqrt{a^{2}-x^{2}}\, dx =\frac{x}{2}\sqrt{a^{2}-x^{2}}+\frac{a^{2}}{2}sin^{-1}\frac{x}{a}+c$

(xv) $\large \int \sqrt{x^{2}+a^{2}}\, dx =\frac{x}{2}\sqrt{x^{2}+a^{2}}+\frac{a^{2}}{2}log\left | x+ \sqrt{x^{2}+a^{2}} \right |+c$

(xvi) $\large \int \sqrt{x^{2}-a^{2}}\, dx =\frac{x}{2}\sqrt{x^{2}-a^{2}}-\frac{a^{2}}{2}log\left | x+ \sqrt{x^{2}-a^{2}} \right |+c$

Walli’s Formula of Integration

If m and n be integers, then

(i) $\large \int_{0}^{\frac{\pi }{2}}sin^{m}xcos^{n}xdx=\left\{\begin{matrix} \frac{(m-1)(m-2)....(1\, or\, 2).(n-1)(n-2).....(1\, or\, 2)}{(m+n)(m+n-2).....(1\, or\, 2)}\frac{\pi }{2}\, \, where\, m, n \, are \, even\, integers \\ \frac{(m-1)(m-3)....(1\, or\, 2).(n-1)(n-3).....(1\, or\, 2)}{(m+n)(m+n-2).....(1\, or\, 2)}\, \, where\, m, n \, are \, odd\, integers \end{matrix}\right.$

(ii) $\large \int_{0}^{\frac{\pi }{2}}sin^{n}xdx=\int_{0}^{\frac{\pi }{2}}cos^{n}xdx=\left\{\begin{matrix} \frac{(n-1)(n-3)(n-5).... 2}{(n)(n-2)(n-4)......,3}\, \, where \, \, n \, is \, odd \\ \frac{(n-1)(n-3)(n-5).... 3 .1}{(n)(n-2)(n-4).......,4 .2}\frac{\pi }{2}\, \, where \, n \, is \, even \end{matrix}\right.$

Class 12 integration formulas PDF

File Name: list-of-basic-integrals.pdf

File Name: extension-formulae-of-standard-integral-forms.pdf

You might also be interested in:

हमारा YouTube Channel Subscribe करें !

Bina singh

View all posts by Bina singh →

Leave a Reply Cancel reply