BODMAS Questions for class 5
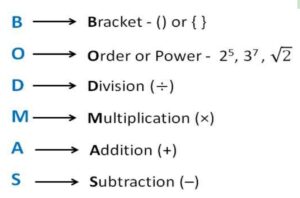
The abbreviation BODMAS rule is used to remember the procedures to be carried out in the right order while solving mathematical expressions. BODMAS stands for Brackets, Orders or Powers, Division, Multiplication, Addition, and Subtraction. Therefore, in BODMAS, the orders or exponents are given the second choice. We then carry out the arithmetic operations. A variant of BODMAS that is used in some regions is PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction).
The BODMAS rule states that if an expression involves brackets ((), {}, []), we must first solve or simplify the bracket before moving on to the left-to-right operations of division, multiplication, addition, and subtraction. A wrong answer will be obtained if the problem is solved incorrectly.
The following questions on simplifications has different types of questions that can be practiced by the students to get more ideas to simplify the expressions.
Contents
Simple BODMAS QUESTIONS for class 5 with answers (Solved examples)
(i) 5 x 50 +57 -57 ÷ 57
Sol. 5 x 50 +57 -57 ÷ 57= 5 x 50 +57 -1 (performing division first)
=250+57-1 ( performing multiplication)
= 307-1 (performing addition )
= 306
(ii) 4+4+4+4 ÷ 4
Sol. 4+4+4+4÷ 4 =4+4+4+1 (solving division)
= 13
(iii) 80+[20 x { 20-(10 ÷5) }]
Sol. 80+[20 x { 20-(10 ÷5) }] = 80+[20 x { 20-2 }] (Solving the small brackets)
=80+[20 x 18] (removing braces)
= 80+360 ( performing multiplication)
=440
(iv) 10 x 10 +[400 ÷ {100-(50-
Sol. 10 x 10 +[400 ÷ {100-(50-)}] = 10 x 10 +[400 ÷ {100-(50-30)}] (removing bar)
= 10 x 10 +[400 ÷ {100-20}] (removing small brackets)
= 10 x 10 +[400 ÷ 80] ( removing braces)
= 10 x 10 +5 (removing big brackets and performing division)
= 100+5 (performing multiplication)
=105
(v) [{64-(12+13)}÷3]+15
Sol. [{64-(12+13)}÷3]+15 = [{64-25}÷3]+15 (removing small brackets)
=[39÷3]+15 (removing braces)
= 13+15 (performing division)
=28
(vi) 7614 +832 x 48 ÷ 16- 8
Sol. 7614 +832 x 48 ÷ 16- 8 =7614 +832 x 3- 8 (performing division)
=7614 +2496- 8 ( performing multiplication)
= 10110-8
= 10102
(vii) 12- [20 ÷{8-2(9-5-2)}]
Sol. 12- [20 ÷{8-2(9-5-2)}] =12- [20 ÷{8-2 x 2}] (solving expression in small brackets)
=12- [20 ÷{8-4}]
=12- [20 ÷4] (removing braces)
= 12 -5 (removing big brackets)
=7
(viii) 25-[16-{5+18÷(4-)}]
Sol. (viii) 25-[16-{5+18÷(4-)}]=25-[16-{5+18÷(4-2)}] (removing bar)
= 25-[16-{5+18÷2}] (removing small brackets)
= 25-[16-{5+9}] (solving division inside curly braces)
=25-[16-14]
=25-2
=23
(ix) 15-[16-{12+21÷(9-2)}]
Sol. 15-[16-{12+21÷(9-2)}]=15-[16-{12+21÷7}]
=15-[16-{12+3}]
=15-[16-15]
=15-1
=14
(x)
Sol.
(xi)
Solution :
= 118-9
=109
(xii) Evaluate: {15 × 32 ÷ 2 × 5} ÷ 75
Sol. {15 × 32 ÷ 2 × 5} ÷ 75 = {15 × 16 × 5} ÷ 75
= {1200} ÷ 75
= 16
(xiii)
Sol: Start by solving the innermost bar bracket
=
=1
(xiv)
Solution : =
=
=
=
‘BODMAS’ Simplification Questions for class 5 (UNSOLVED Examples)
(Simple BODMAS questions with answers)
(i) 12+12 ÷ 3
(ii) 16 x 10÷ 2
(iii) 24-8 x 2
(iv) 32 ÷ 8+4 x 6-2
(v) 100-72 ÷ 8+4 x 3
(vi) 30+75 ÷ 15 x 4 -18
(vii) 8 x 6 +24 ÷ 6- 18
(viii) 56- 36 ÷ 4 x 2 +7
(ix) 56 ÷ 14 x 3 -10 ÷5 +1
(x) 198-42 x 15 ÷ 5 +6
(xi) 105 x 14 ÷ 7 +6 -1
(xii) 17+34 ÷ 17 x 5 -20
(xiii) 289+ 153 ÷ 17-8 x 19
(xiv) 7614+832 x 48 ÷16-8
(xv) 7823-128 ÷16 of 4 -3973
(xvi)
Ans. (i) 16 (ii) 80 (iii) 8 (iv) 26 (v) 103 (vi) 32 (vii) 34 (viii) 47 (ix) 11 (x) 78 (xi) 215 (xii) 7 (xiii) 146
(xiv) 10102 (xv) 3848 (xvi) 1
BODMAS Questions for class 5 PDF DOWNLOAD
BODMAS QUIZ FOR CLASS 5
Click ‘Start Quiz’ to begin!
You might also be interested in:
- PEMDAS rule
- simplification
- BODMAS problems
- Order of Operations
- simplification tricks
- Unitary method questions for class 5

